Square Root Of 196 By Prime Factorization

Thew following steps will be useful to find square root of a number by prime factorization.

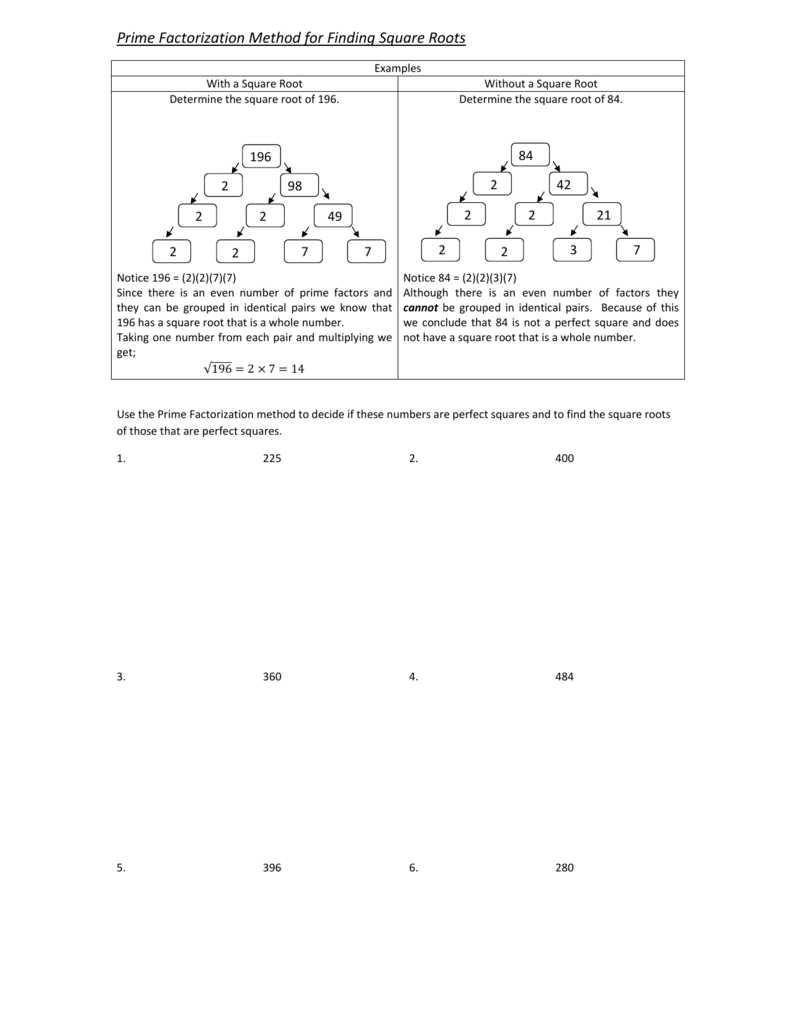
Square root of 196 by prime factorization.

Ii inside the square root for every two same numbers multiplied one number can be taken out of the square root. If these factors are further restricted to prime numbers the process is called prime factorization. Square root by prime factorization method example 1 find the square root. If we make the pair of the prime factors we see that the prime factor 5 is not in the pair.

The number 1 is not a prime number but a divider for every natural number. Cubed root of 196. When the numbers are sufficiently large no efficient non quantum integer factorization algorithm is known. Prime factors of 196.

To learn more about squares and square roots enrol in our full course now. The product obtained in step v is the required square root. Find the product of factors obtained in step iv. Factorization in a prime factors tree for the first 5000 prime numbers this calculator indicates the index of the prime number.

So the square root of 441 441 21. Let us find the square root of 180. It is often taken as the smallest natural number however some authors include the natural numbers from zero. Notice 196 2 2 7 7 since there is an even number of prime factors and they can be grouped in identical pairs we know that 196 has a square root that is a whole number.

Take one factor from each pair. Https bit ly exponentsandpowersg8 in this video we will learn. I decompose the number inside the square root into prime factors. The n th prime number is denoted as prime n so prime 1 2 prime 2 3 prime 3 5 and so on.

The prime factorization of 180 is 180 2 2 3 3 5. Your prime factorization is the empty product with 0 factors which is defined as having a value of 1. 0 00 how to fin. With a square root without a square root determine the square root of 196.

Since the number is a perfect square you will be able to make an exact number of pairs of prime factors. In 2019 fabrice boudot pierrick gaudry aurore guillevic nadia.